1. Hilt, V., E. Noel, C. Shen, and A. Abdelal. Design considerations for Session Initiation Protocol (SIP) overload control. 2011. Internet Engineering Task Force RFC- 6357. Available at: http://tools.ietf.org/html/rfc6357 (accessed October 1, 2013).
2. Hilt, V., J. Rosenberg, H. Schulzrinne, et al. SIP: Session Initiation Protocol. 2002. Internet Engineering Task Force RFC-3261. Available at: http:// tools.ietf.org/html/rfc3261 (accessed October 1, 2013).
3. Rosenberg, J. Requirements for management of overload in the Session Initiation Protocol. 2008. Internet Engineering Task Force RFC-5390. Available at: http:// tools.ietf.org/html/rfc5390 (accessed October 1, 2013).
4. Gurbani, V., V. Hilt, and H. Schulzrinne. Session Initiation Protocol overload control. 2013. Internet Engineering Task Force Draft. Available at: http://tools.ietf.org/pdf/draft-ietf-soc-overload- control-13.pdf (accessed October 1, 2013).
5. Noel, E., and P.M. Williams. Session Initiation Protocol rate control. 2013. Internet Engineering Task Force Draft. Available at: http://tools.ietf.org/pdf/draft-ietf- soc-overload-rate-control-05.pdf (accessed October 1, 2013).
6. ITU-T Recommendation Q.704: Signalling system No. 7 — Message Transfer Part, Signalling network functions and messages. 1996. Available at: http:// www.itu.int/rec/T-REC-Q.704-199607-I/en (accessed October 1, 2013).
7. Abaev, P., Yu. Gaidamaka, and K. Samouylov. 2011. Gisterezisnoe upravlenie signal’noy najruzkoy v seti SIPserverov [Hysteretic overboad control in a SIP signaling network]. Vestnik RUDN. SeriyaMatematika, Informatika, Fizika [Bulletin of Peoples’ Friendship University of Russia. Ser. Mathematics. Informatics. Physics] 4:55–73.
8. Abaev, P., Yu. Gaidamaka, A. Pechinkin, R. Razumchik, and S. Shorgin. 2012. Simulation of overload control in SIP server networks. 26th Conference (European) on Modelling and Simulation ECMS Proceedings. Koblenz. 533–39.
9. Abaev, P., Yu. Gaidamaka, and K. Samouylov. 2012. Queuing model for loss-based overload control in a SIP server using a hysteretic technique. Internet of things, smart spaces, and next generation networking. Eds. S. Andreev, S. Balandin, and Ye. Koucheryavy. Lecture notes in computer science ser. Heidelberg: Springer-Verlag. 7469:371– 78.
10. Gebhart, R. F. 1967. A queuing process with bilevel hysteretic service-rate control. Nav. Res. Logist. Q. 14:55–68.
11. Krasnoselskii, M., and A. Pokrovskii. 1989. Systems with hysteresis. Berlin–Heidelberg–New York–London– Paris–Tokio: Springer-Verlag. 410 p.
12. Yum, T., and H. Yen. 1983. Design algorithm for a hysteresis buffer congestion control strategy. IEEE Conference (International) on Communications Proceedings. 499–503.
13. Brown, P., P. Chemouil, and B. Delosme. 1984. A congestion control policy for signalling networks. 7th IeCC Proceedings. 717–24.
14. Golubchik, L., and J. Lui. 1997. Bounding of performance measures for a threshold-based queueing system with hysteresis. Newsl. ACM SIGMETRICS Performance Evaluation Rev. 25(1):147–57.
15. Sindal, R., and S. Tokekar. 2008. Modeling and analysis of voice/data call admission control scheme in CDMA cellular network for variation in soft handoff threshold parameters. 16th IEEE Conference (International) on Networks (ICON 2008) Proceedings. 1–6.
16. Zhernovyi, K., and Yu. Zhernovyi. 2012. Sistema 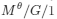  s gisterezisnym pereklyucheniem intensivnosti obsluzhivaniya [The system 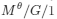  with a hysteretic switching intensity of service]. Information Processes 12(3):176–90.
17. Takagi, H. 1985. Analysis of a finite-capacity M/G/1 queue with a resume level. Perform. Evaluation 5:197– 203.
18. Benaboud, H., and N. Mikou. 2002. Analysis by queuing model of multi-threshold mechanism in ATM switches. 5th IEEE Conference (International) on High Speed Networks and Multimedia Communications (HSNMC 2002) Proceedings. 147–51.
19. Dshalalow, J.H. 1997. Queueing systems with state dependent parameters. Frontiers in queueing: Models and applications in science and engineering. Ed. J.H. Dshalalow. Probability and stochastic ser. CRC Press. 61–116.
20. Bekker, R. 2009. Queues with Levy input and hysteretic control. Queueing Syst. 63(1):281–99.
21. Roughan, M., and C. Pearce. 2000. A martingale analysis of hysteretic overload control. Adv. Perform. Anal. J. Teletraffic Theory Performance Anal. Communication Syst. Networks. 3(1):1–30.
22. Abaev, P., Yu.Gaidamaka, andK. Samouylov. 2012.Modeling of hysteretic signalling load control in next generation networks. Internet of things, smart spaces, and next generation networking. Eds. S. Andreev, S. Balandin, and Ye. Koucheryavy. — Lecture notes in computer science ser. Heidelberg: Springer-Verlag. 7469:440–52.
23. Abaev, P., and R. Razumchik. 2012. Modelirovanie raboty SIP servera s pomoshchyu sistemy massovogo obsluzhivaniya s gisterezisom i progulkami v diskretnom vremeni [Modeling of SIP-server with hysteric overload control as discrete time queueing system]. T-Comm — Telekommunikacii i transport [T-Comm—Telecommunications and Transport] 7:5–8.
24. Gaidamaka, Yu., K. Samouylov, and E.Sopin. 2012. Model’  odnoy sistemy massovogo obsluzhivaniya tipa M/G/1 s gisterezisnym upravleniem vkhodyashchim potokom [On queueing system of M|G|1 type with hysteretic input flow control]. T-Comm — Telekommunikacii i transport [T-Comm— Telecommunications and Transport] 7:60–62.
25. Abaev, P., A. Pechinkin, and R. Razumchik. 2012. On analytical model for optimal SIP server hop-by-hop overload control. 4th Congress (International) on Ultra Modern Telecommunications and Control Systems Proceedings. IEEE. 299–304. doi:10.1109/ICUMT.2012.6459680.
26. Abaev, P., A. Pechinkin, and R. Razumchik. 2013. Analysis of queueing system with constant service time for SIP server hop-by-hop overload control. Modern Probab. Meth. Anal. Telecommunication Networks Communications Computer Information Sci. 356: 1–10. doi:10.1007/978-3- 642-35980-4 1.
27. Abaev, P., A. Pechinkin, and R. Razumchik. 2013. On mean return time in queueing system with constant service time and bi-level hysteric policy. Modern Probab. Meth. Anal. Telecommunication Networks Communications Computer Information Sci. 356:11–19. doi:10.1007/978-3- 642-35980-4 2.
28. Abaev, P., Yu. Gaidamaka,K. Samouylov, and S. Shorgin. 2013. Design and software architecture of SIP server for overload control simulation. 27th Conference (European) on Modelling and Simulation Proceedings. Aalesund, Norway. 533–39. doi:10.7148/2013-0580.
29. Pechinkin, A., and R. Razumchik. 2013. Approach for analysis of finite M2/M2/1/R with hysteric policy for SIP server hop-by-hop overload control. 27th Conference (European) on Modelling and Simulation Proceedings. Aalesund, Norway. 573–79. doi:10.7148/2013.
30. Shorgin, S., K. Samouylov, Yu. Gaidamaka, and Sh. Etezov. 2013. Polling systemwith threshold control formodeling of SIP server under overload. 18th Conference (International) on Systems Science Proceedings.Wroclaw: Springer International Publs. 240:97–107.doi:10.1007/978-3-319- 01857-7 10.
31. Gaidamaka, Yu. 2013. Model’ s porogovym upravleniem nagruzkoy dlya analiza serverov protokola SIP v rezhime peregruzok [On model with threshold load control for SIP server overload analysis]. Avtomatika i vychislitel’naya tekhnika [Automatic Control and Computer Sciences] 4:65– 75.
32. Abaev, P., Yu. Gaidamaka, K. Samouylov, A. Pechinkin, R. Razumchik, and S. Shorgin. 2014 (in press). Hysteretic control technique for overload problem solution in network of SIP servers. Comput. Inform. 33(1).
33. MathWorks — MATLAB and Simulink for Technical Computing. Available at: http://www.mathworks.com (accessed October 1, 2013).
34. Wolfram Mathematica: Programmnoe obespechenie dlya tekhnicheskikh vychisleniy [Software for numerical computations]. Available at: http://www. wolfram.com/mathematica (accessed October 1, 2013).
35. Maple — Technical Computing Software for Engineers, Mathematicians, Scientists, Instructors and Students. Available at: http://www.maplesoft.com/ products/maple (accessed October 1, 2013).
36. Bocharov, P.P., C.D’Apice, A. V. Pechinkin, and S. Salerno. 2004. Queueing theory. Utrecht, Boston: VSP. 446 p.
37. Kempa, W. 2012. On time to buffer saturation in a GI/M/1/N-type queue. Int. J. Adv. Telecommunications Electrotechnics Signals Syst. 1(2-3):60–66.

Title

THE DISTRIBUTION OF THE RETURN TIME FROM THE SET OF OVERLOAD STATES TO THE SET OF NORMAL LOAD STATES IN A SYSTEM WITH HYSTERETIC LOAD CONTROL

Journal

Informatics and Applications
December 2013, Volume 7, Issue 4, pp 20-33

Cover Date

2013-12-31

DOI

10.14357/19922264130403

Print ISSN

1992-2264

Publisher

Institute of Informatics Problems, Russian Academy of Sciences

Additional Links

• Editorial Board
• About This Journal
• Manuscript Submission

Key words

SIP-server overload; queueing system; hysteretic load control; return time to normal load states; Laplace–Stieltjes transform; distribution function

Authors

Yu. V. Gaidamaka, A. V. Pechinkin, R. V. Razumchik, A.K. Samuylov, K. E. Samouylov, I.A. Sokolov, E. S. Sopin, and S. Ya. Shorgin

Author Affiliations

   Peoples’ Friendship University of Russia, Moscow, Russia
   Institute of Informatics Problems, Russian Academy of Sciences, Moscow, Russia